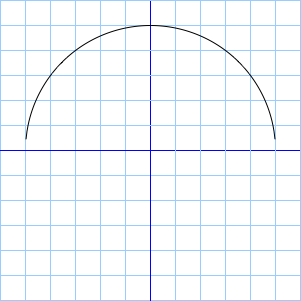
Once there was a variable, and the variable's name was x. Ze was used in a function named f. In this function, x was squared, subtracted from one, and then square rooted. A beautiful graph the function made – a semicircle, like a rainbow. One could almost see the pot of gold at f(±1). Suddenly the graph was filled in. x noticed that there was an odd line, curved at the end, before f, with numbers on it. Suddenly, x felt an odd sensation – f was disappearing, being simplified away. What was left was half a pi – a delicious-looking rhubarb pie, to be specific. Not just any rhubarb pie, no, this was a Bee-Bop-a-Re-Bop rhubarb pie, the kind that supports Prairie Home Companion. x looked back on zir life, wondering if there was a long chain of events leading to a bad situation which would warrant said pie. Ze remembered the time when they took the derivative of f. They separated out the 1 + x2 from the square root, called it the "chain rule" or something. Perhaps that could be the chain of events? g'(x)f'(g(x))? Or not. That just seemed to end up in... nothing. They were back where they started. Nothing ever changed... except for x's value. x looked back... ze liked being zero, then the function was nice and positive, even if only positive one. They never seemed to get higher than that. So x ate the half pi. The end.
(Author's note: Integral from -1 to 1 of the square root of 1 - x2 is, indeed, π÷2.)